
Unlike 4th grade, 5th graders will now be expected to add and subtract fractions with unlike denominators.
While at first this may feel like you have 5 oranges and are asked to subtract 3 apples, using models will help students find common denominators and add and subtract fractions.
Easy as fruit pie!
Adding Fractions With Unlike Denominators
Egg Cartons are great for introducing adding with unlike denominators, as all fractions can easily be converted to twelfths.
3/4 of an egg carton is 9 eggs. 5/6 of an egg carton is 10 eggs. 9 eggs + 10 eggs = 19 eggs. Substitute “eggs” with “twelfths” and suddenly it all makes sense. 9/12 + 10/12 = 19/12. Using an egg carton will also help students convert that improper fraction to a mixed number. 19/12 = 1 7/12.
This model shows 2/6 + 1/4 using a FREE PRINTABLE worksheet you can download and use with your students. Students start by drawing the two fractions, showing the conversion to twelfths, then solving the problem. They can check their work using the pictures to make sure they’re answering the questions correctly.
Subtracting Fractions With Unlike Denominators

Start with egg cartons again. Let’s say we start with 5/6 and are asked to subtract 1/2. Students can fill an egg carton 5/6 (10 eggs, or 10/12). Watch out for the common mistake of removing half of 5/6, or half of the 10 eggs. Remind students that 1/2 of the carton is 6 eggs, so they will need to remove 6 eggs.

You can also use the free worksheet to model fraction subtraction. In this example, a student started with 1 1/2 (18 eggs), modeled 3/4 below, then crossed off 9 eggs to subtract 3/4 from the top.
Download the Adding & Subtracting Fractions Egg Carton Math Freebie
First, fill out the form below. After you confirm your subscription, the free resources will be sent to your inbox!
Whizzing Through Word Problems
Step 1: Slow down! Read and reread the problem until it makes sense. Be able to tell the problem in your own words.
Step 2: Draw a picture to show your thinking. Think about what your answer should be. Less than a whole, or more? Close to a half, or close to a whole? Having an idea of what the answer should be will help catch mistakes.
Step 3: Write an equation and solve the problem.
Step 4: Check your work and make sure your answer makes sense. Is your answer near the estimate you made in the beginning? Did you answer the question that was asked?




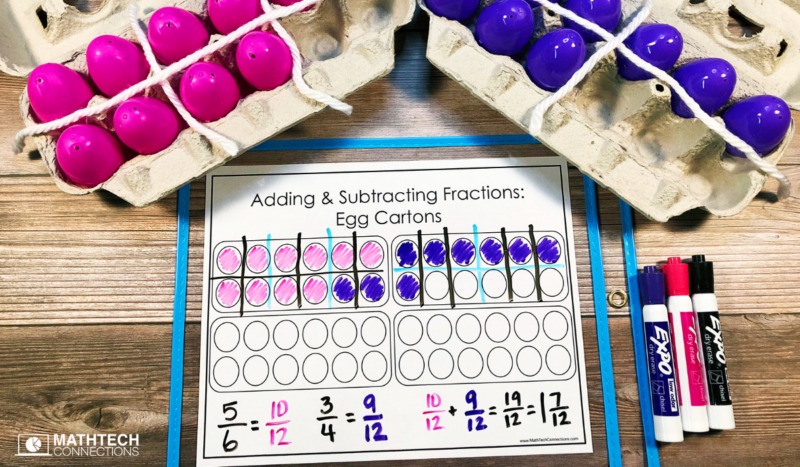 3/4 of an egg carton is 9 eggs. 5/6 of an egg carton is 10 eggs. 9 eggs + 10 eggs = 19 eggs. Substitute “eggs” with “twelfths” and suddenly it all makes sense. 9/12 + 10/12 = 19/12. Using an egg carton will also help students convert that improper fraction to a mixed number. 19/12 = 1 7/12.
3/4 of an egg carton is 9 eggs. 5/6 of an egg carton is 10 eggs. 9 eggs + 10 eggs = 19 eggs. Substitute “eggs” with “twelfths” and suddenly it all makes sense. 9/12 + 10/12 = 19/12. Using an egg carton will also help students convert that improper fraction to a mixed number. 19/12 = 1 7/12. This model shows 2/6 + 1/4 using a FREE PRINTABLE worksheet you can download and use with your students. Students start by drawing the two fractions, showing the conversion to twelfths, then solving the problem. They can check their work using the pictures to make sure they’re answering the questions correctly.
This model shows 2/6 + 1/4 using a FREE PRINTABLE worksheet you can download and use with your students. Students start by drawing the two fractions, showing the conversion to twelfths, then solving the problem. They can check their work using the pictures to make sure they’re answering the questions correctly.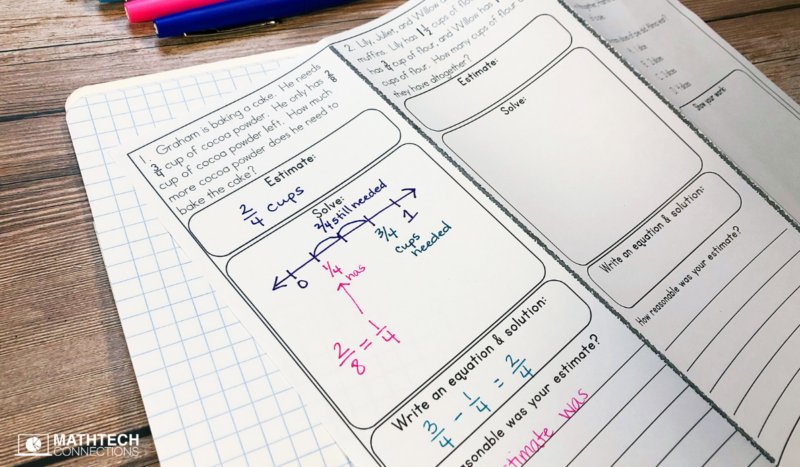












Leave a Comment